Applying optical reciprocity to the problem of dipolar emission near a planar interface, we can obtain the radiation pattern (intensity vs angle) by modelling the near-field at the dipole location for plane-wave illumination at a range of incident angles.

library(planar)
library(ggplot2)
require(reshape2)

Dipole in a vacuum

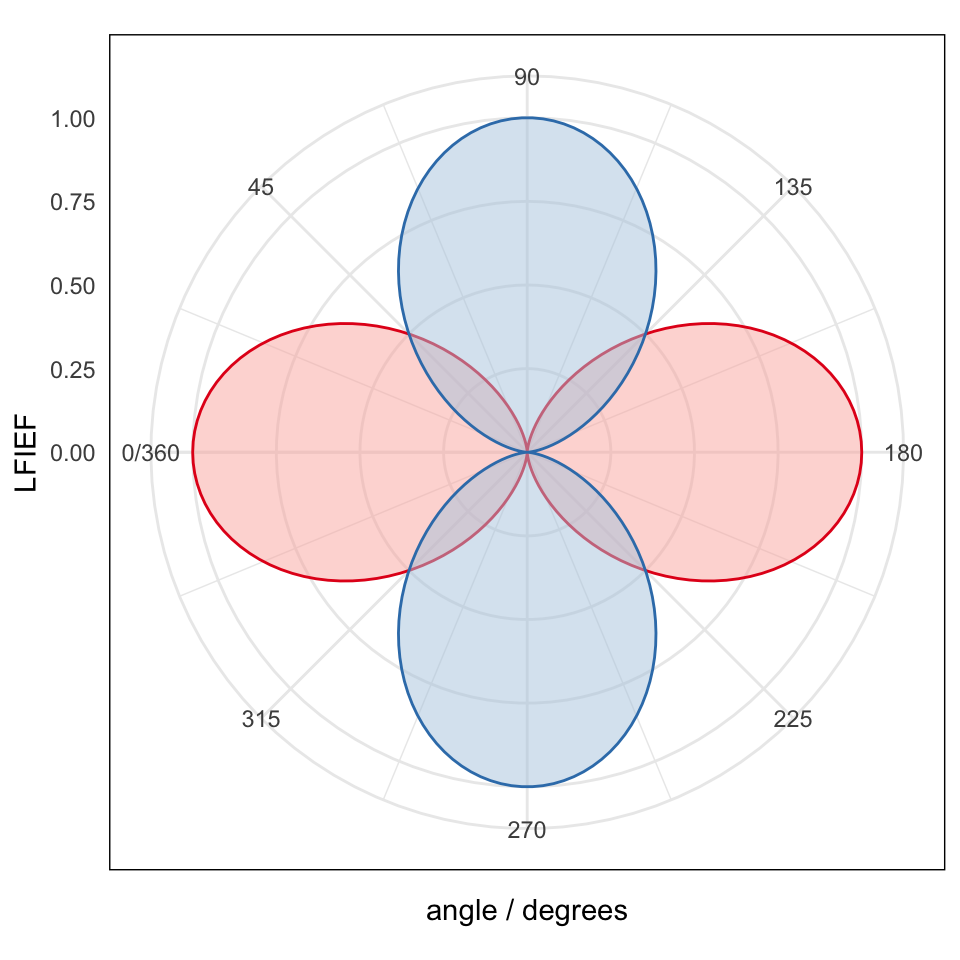
We first confirm that the dipolar emission in a vacuum follows the expected sin^2 pattern. We define a dummy simulation (no actual interface),

## radiation pattern of a dipole in a vacuum
## parallel and perpendicular orientations, p- polarisation

## from left to right
## incidence air | air

stack <- list(wavelength=632.8,
             angle = seq(0, pi, length=100), 
             epsilon = list(1.0^2, 1.0^2),
             thickness = c(0, 0),
             d = 1,
             polarisation = 'p')
## simulation (side of incidence is irrelevant)
M <- do.call(multilayer, stack)
str(M)

## List of 15
##  $ wavelength  : num 633
##  $ k0          : num 0.00993
##  $ angle       : num [1:100] 0 0.0317 0.0635 0.0952 0.1269 ...
##  $ q           : num [1:100] 0 0.0317 0.0634 0.0951 0.1266 ...
##  $ reflection  : cplx [1:100] 0+0i 0+0i 0+0i ...
##  $ transmission: cplx [1:100] 1+0i 1+0i 1+0i ...
##  $ R           : num [1:100] 0 0 0 0 0 0 0 0 0 0 ...
##  $ T           : num [1:100] 1 1 1 1 1 1 1 1 1 1 ...
##  $ A           : num [1:100] 0 0 0 0 0 0 0 0 0 0 ...
##  $ dist        :List of 2
##   ..$ : num -1
##   ..$ : num 1
##  $ fields      :List of 4
##   ..$ Eix.E1 : cplx [1, 1:100, 1:2] 1+0i 0.999+0i 0.998+0i ...
##   ..$ Epix.E1: cplx [1, 1:100, 1:2] 0+0i 0+0i 0+0i ...
##   ..$ Eiz.E1 : cplx [1, 1:100, 1:2] 0+0i -0.0317+0i -0.0634+0i ...
##   ..$ Epiz.E1: cplx [1, 1:100, 1:2] 0+0i 0+0i 0+0i ...
##  $ Ml.perp     :List of 1
##   ..$ : num [1:100] 0 0.00101 0.00402 0.00904 0.01603 ...
##  $ Ml.par      :List of 1
##   ..$ : num [1:100] 1 0.999 0.996 0.991 0.984 ...
##  $ Mr.perp     :List of 1
##   ..$ : num [1:100] 0 0.00101 0.00402 0.00904 0.01603 ...
##  $ Mr.par      :List of 1
##   ..$ : num [1:100] 1 0.999 0.996 0.991 0.984 ...

# combine results of front vs back illumination simulations (identical here, but anyway...)
combined <- data.frame(angle = c(stack$angle, stack$angle + pi) * 180/pi,
                      parallel = c(M$Mr.par[[1]], M$Ml.par[[1]]),
                      perpendicular = c(M$Mr.perp[[1]], M$Ml.perp[[1]]),
                      side = gl(2, length(stack$angle),
                                labels=c("front", "back")))

## basic plot
qplot(angle, perpendicular, colour=side, data=combined, geom="line")

## polar plot with two variables
m <- melt(combined, id=c("angle", "side"))

ggplot(m, aes(angle, value)) +
  geom_polygon(aes(fill=variable, colour=variable), alpha=0.5)  +
  scale_x_continuous(breaks = seq(0, 360, by = 45),
                     limits = c(0, 360), expand = c(0, 0)) +
  coord_polar(start=-pi/2) +
  scale_fill_brewer(palette = "Pastel1") +
  scale_colour_brewer(palette = "Set1") +
  labs(x = "angle / degrees", y = "LFIEF", 
       fill = "Orientation") +
  guides(fill="none", colour="none")

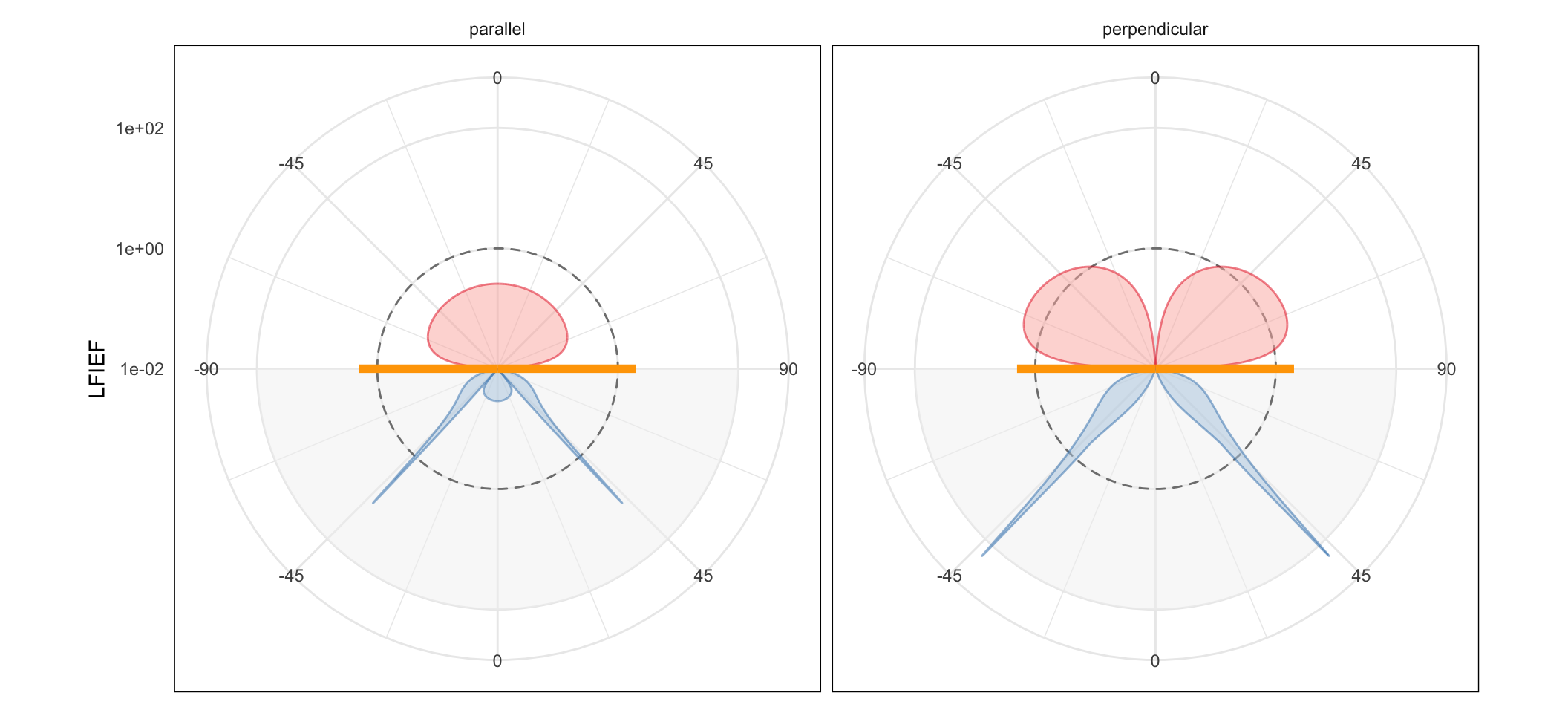
Dipole near a thin gold film (Kretschmann configuration)

In the Kretschmann configuration, molecules situated on the air side will radiate predominantly in a narrow cone of angles, associated with the excitation of surface plasmon-polaritons (SPPs) radiating into the glass substrate. By reciprocity, the field enhancement experienced by a dipole near the metal layer is also obtained for a narrow range of angles where conservation of in-plane momentum between the incident light and the SPPs is satisfied.

## from left to right
## incidence air | metal | glass

back <- list(wavelength=632.8,
             angle = seq(-pi/2, pi/2, length=1e4)[-c(1, 1e4)], 
             epsilon = list(1.52^2, epsAg(632.8)$epsilon, 1.0^2),
             thickness = c(0, 50, 0),
             d = 1,
             polarisation = 'p')

## incidence glass | metal | air
front <- invert_stack(back)

## simulation
M.front <- do.call(multilayer, front)
M.back <- do.call(multilayer, back)

# redefine angles to span 0 - 360 degrees
angle <- c(front$angle + pi/2, back$angle + 3*pi/2) * 180 / pi

## look at field in first and last media, respectively
last <- length(M.back$Mr.par)
intensity.par = c(M.front$Ml.par[[1]] , M.back$Mr.par[[last]])
intensity.perp = c(M.front$Ml.perp[[1]] , M.back$Mr.perp[[last]])

combined <- data.frame(angle=angle, parallel=intensity.par,
                       perpendicular=intensity.perp,
                       side = gl(2, length(back$angle),
                                labels=c("front", "back")))

## polar plot with two variables
m <- melt(combined, id=c("angle", "side"))

# custom labels for polar plot
mylabels <- c(-90,-45,0,45,90,45,0,-45) 

ggplot(m, aes(angle, value)) +
  facet_wrap(~variable, ncol=2) +
  annotate("rect", xmin=180, xmax=360, ymin=1e-2, ymax=100,
           fill="grey95", alpha=0.5) +
  annotate("rect", xmin=0, xmax=360, ymin=1e-2, ymax=1,
           fill=NA, colour="grey50", lty="dashed") +
  geom_polygon(aes(fill=side), alpha=0.5, colour=NA)  +
  geom_line(aes(colour=side), alpha=0.5)  +
  scale_x_continuous(breaks = seq(0, 360-45, by = 45), labels=mylabels,
                     limits = c(0, 360), expand = c(0, 0)) +
  scale_y_log10(lim=c(1e-2, 200)) +
  coord_polar(start=3*pi/2) +
  annotate("segment", x=360, xend=360, y=0, yend=2, colour="orange", size=2) +
  annotate("segment", x=180, xend=180, y=0, yend=2, colour="orange", size=2) +
  scale_fill_brewer(palette = "Pastel1") +
  scale_colour_brewer(palette = "Set1") +
  labs(x = NULL, y = "LFIEF", fill = "side",linetype="Orientation") +
  guides(fill="none",colour="none")